PYTHAGORAS (c.560 – c.480 BCE)

Sixth Century BCE – Greece

In a right-angled triangle, the square on the hypotenuse is the sum of the squares on the other two sides

The Theorem may also be written as a general law:  a² + b² = c²  where c is the length of the hypotenuse of a right-angled triangle, and a and b the lengths of the other two sides. Pythagoras’ theorem is a starting point for trigonometry, which has many practical applications such as calculating the height of mountains and measuring distances.

c.525 BCE – Pythagoras taken prisoner by the Babylonians

c.518 BCE – establishes his own academy at Croton (now Crotone) in southern Italy

c.500 BCE – Pythagoras moves to Metapontum

Pythagoras was the first to prove the relationship between the sides of a right-angled triangle, but he did not discover it – it was known to Babylonians for nearly 1000 years before him.

PYTHAGORAS

His disciples, members of the semi-religious, philosophical school he founded, may have actually found many of the mathematical discoveries credited to Pythagoras. The inner circle of followers were known as mathematikoi and, unusually for the time, included women among its membership. An outer circle, the akousmatics, lived in their own homes and came in to the school by day.

Of the five key beliefs the Pythagoreans held, the idea that ‘all is number’ was dominant; the belief that reality at its fundamental level is mathematical and that all physical things like musical scales, or the spherical earth and its companions the stars and the universe, are mathematically related. Pythagoras was responsible for the widely held Greek belief that real knowledge had to be like mathematics – universal, permanent, obtained by pure thought and uncontaminated by the senses.

Because of the reverence with which the originator of the Pythagoreans was treated by his followers and biographers, it is difficult to discern legend from fact, such as the notion that he was the first to offer a three-part argument that the shape of the Earth is spherical:
The field of stars changes with the latitude of the observer; the mast of a ship comes into view before its hull as the ship approaches the shore from a distance; and the shadow of the Earth cast on the moon during a lunar eclipse is always round.

After Pythagoras, the idea of a ‘perfect’ mathematical interrelation between a globe moving in circles and the stars behaving similarly in a spherical universe inspired later Greek scholars, including ARISTOTLE, to seek and ultimately find physical and mathematical evidence to reinforce the theory of the world as an orb.

Attributed to the Pythagoreans is the discovery that simple whole number ratios of string lengths produce harmonious tones when plucked, probably the first time a physical law had been mathematically expressed.

Numerous other discoveries such as ‘the sum of a triangle’s angles is the equal to two right angles’ and ‘the sum of the interior angles in a polygon of n-sides is equal to 2n-4 right angles’ were made. They also discovered irrational numbers, from the realisation that the square root of two cannot be expressed as a perfect fraction. This was a major blow to the Pythagorean idea of perfection and according to some, attempts were made to try to conceal the discovery.

PLATONIC SOLIDS

To the Pythagoreans, the fifth polyhedron had monumental significance. Outnumbering by one the number of recognized elements, the dodecahedron was considered to represent the shape of the universe. 
A omerta, or code of silence, was imposed regarding the dodecahedron and divulging this secret to outsiders could mean a death penalty.

NEXT

THE PLATONIC SOLIDS

The properties of solid figures have kept mathematicians occupied for centuries. Regular polyhedra are formed from regular polygons such as squares or triangles and mathematicians have failed to find any more than five of them.

 

“THE PLATONIC SOLIDS – The regular polyhedron is defined as a three-dimensional solid comprising regular polygons for its surfaces – and with all its surfaces, edges and vertices identical. The five regular polyhedra are the tetrahedron (four triangular faces), the cube (six square faces), the octahedron (eight triangular faces), the dodecahedron (twelve pentagonal faces) and the icosahedron (twenty triangular faces).”

 
 
 
 

Although they were defined by Pythagoras two hundred years before PLATO was born, they are known collectively as the platonic solids, named in honour of Plato by the geometer Euclid.

MAIN INDEX

 

Related articles

• the-golden-ratio-and-astronomy (blogs.stsci.edu)

EUCLID (c.330 – c.260 BCE)

Fourth century BCE – Alexandria, Egypt

EUCLID

1. A straight line can be drawn between any two points

2. A straight line can be extended indefinitely in either direction

3. A circle can be drawn with any given centre and radius

4. All right angles are equal

5. If two lines are drawn which intersect a third in such a way that the sum of the inner angles on one side is less than two right angles, then the two lines will eventually meet (or, parallel lines never meet)

These five postulates form the basis of Euclidean geometry. Many mathematicians do not consider the fifth postulate (or parallel postulate) as a true postulate, but rather as a theorem that can be derived from the first four postulates. This ‘parallel’ axiom means that if a point lies outside a straight line, then only one straight line can be drawn through the point that never meets the other line in that plane.

The ideas of earlier Greek mathematicians, such as EUDOXUS, THEAETETUS and PYTHAGORAS are all evident, though much of the systematic proof of theories, as well as other original contributions, was Euclid’s.

The first six of his thirteen volumes were concerned with plane geometry; for example laying out the basic principles of triangles, squares, rectangles and circles; as well as outlining other mathematical cornerstones, including Eudoxus’ theory of proportion. The next four books looked at number theory, including the proof that there is an infinite number of prime numbers. The final three works focused on solid geometry.

Virtually nothing is known about Euclid’s life. He studied in Athens and then worked in Alexandria during the reign of Ptolemy I

Euclid’s approach to his writings was systematic, laying out a set of axioms (truths) at the beginning and constructing each proof of theorem that followed on the basis of proven truths that had gone before.

Elements begins with 23 definitions (such as point, line, circle and right angle), the five postulates and five ‘common notions’. From these foundations Euclid proved 465 theorems.

A postulate (or axiom) claims something is true or is the basis for an argument. A theorem is a proven position, which is a statement with logical constraints.

Euclid’s common notions are not about geometry; they are elegant assertions of logic:

• Two things that are both equal to a third thing are also equal to each other

• If equals are added to equals, the wholes are equal

• If equals are subtracted from equals, the remainders are equal

• Things that coincide with one and other are equal to one and other

• The whole is greater than the part

One of the dilemmas that he presented was how to deal with a cone. It was known that the volume of a cone was one-third of the volume of a cylinder that had the same height and base diameter. He asked if you cut through a cone parallel to its base, would the circle formed on the top section be the same size as that on the bottom of the new, smaller cone?

If it were, then the cone would in fact be a cylinder and clearly that was not true. If they were not equal, then the surface of a cone must consist of a series of steps or indentations.

NON-EUCLIDEAN MATHEMATICS

Janus Bolyai

The essential weakness in Euclidean mathematics lay in its treatment of two- and three- dimensional figures. This was examined in the nineteenth century by the Romanian mathematician Janus Bolyai. He attempted to prove Euclid’s parallel postulate, only to discover that it is in fact unprovable. The postulate means that only one line can be drawn parallel to another through a given point, but if space is curved and multidimensional, many other parallel lines can be drawn. Similarly the angles of a triangle drawn on the surface of a ball add up to more than 180 degrees.
CARL FRIEDRICH GAUSS was perhaps the first to ‘doubt the truth of geometry’ and began to develop a new geometry for curved and multidimensional space. The final and conclusive push came from BERNHARD RIEMANN, who developed Gauss’s ideas on the intrinsic curvature of surfaces.

Riemann argued that we should ignore Euclidean geometry and treat each surface by itself. This had a profound effect on mathematics, removing a priori reasoning and ensuring that any future investigation of the geometric nature of the universe would have to be at least in part, empirical. This provides a mechanism for examinations of multidimensional space using an adaptation of the calculus.

However, the discoveries of the last two hundred years that have shown time and space to be other than Euclidean under certain circumstances should not be seen to undermine Euclid’s achievements.

Moreover, Euclid’s method of establishing basic truths by logic, deductive reasoning, evidence and proof is so powerful that it is regarded as common sense.

TIMELINE

HIPPARCHUS (c.190 – c.125 BCE)

134 BCE – Nicaea, Turkey

Observation of a new star in the constellation Scorpio

The ‘Precession of the Equinoxes’

Hipparchus

By the time Hipparchus was born, astronomy was already an ancient art.

Hipparchus plotted a catalogue of the stars – despite warnings that he was thus guilty of impiety. Comparing his observations with earlier recordings from Babylonia he noted that the celestial pole changed over time.
He speculated that the stars are not fixed as had previously been thought and recorded the positions of 850 stars.

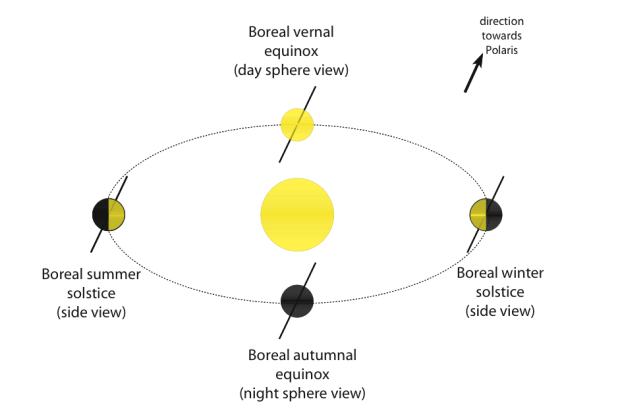
Hipparchus‘ astronomical calculations enabled him to plot the ecliptic, which is the path of the Sun through the sky. The ecliptic is at an angle to the Earth‘s equator, and crosses it at two points, the equinoxes (the astronomical event when the Sun is at zenith over the equator, marking the two occasions during the year when both hemispheres are at right angles to the Sun and day and night are of equal length).

The extreme positions of summer and winter mark the times in the Earth’s orbit where one of the hemispheres is directed towards or away from the Sun.

Solstice
The Sun is furthest away at the solstices.

From his observations, he was able to make calculations on the length of the year.
There are several ways of measuring a year astronomically and Hipparchus measured the ‘tropical year’, the time between equinoxes.

Schematic presentation of a seasonal cycle

Hipparchus puzzled that even though the Sun apparently traveled a circular path, the seasons – the time between the solstices and equinoxes – were not of equal length. Intrigued, he worked out a method of calculating the Sun’s path that would show its exact location on any date.

To facilitate his celestial observations he developed an early version of trigonometry. With no notion of sine, he developed a table of chords which calculated the relationship between the length of a line joining two points on a circle and the corresponding angle at the centre.

By comparing his observations with those noted by Timocharis of Alexandria a century and a half previously, Hipparchus noted that the points at which the equinox occurred seemed to move slowly but consistently from east to west against the backdrop of fixed stars.

We now know that this phenomenon is not caused by a shift in the stars.
Because of gravitational effects, over time the axis through the geographic North and South poles of the Earth points towards different parts of space and of the night sky.
The Earth’s rotation experiences movement caused by a slow change in the direction of the planet’s tilt; the axis of the Earth ‘wobbles’, or traces out a cone, changing the Earth’s orientation as it orbits the Sun.
The shift in the orbital position of the equinoxes relative to the Sun and the change in the seasons is now known as ‘the precession of the equinoxes’, but Hipparchus was basically right.

Hipparchus‘ only large error was to assume, like all those of his time except ARISTARCHUS that the Earth is stationary and that the Sun, moon, planets and stars revolve around it. The fact that the stars are fixed and the Earth is moving makes such a tiny difference to the way the Sun, moon and stars appear to move that Hipparchus was still able to make highly accurate calculations.

These explanations may show how many people become confused by claims that the Earth remains stationary as was believed by the ancients – from our point-of-view on Earth that IS how things could appear.

a) demonstration of precession.

https://www.youtube.com/watch?v=qlVgEoZDjok (copyright)

b) demonstration of the equinoxes, but not of the precession, which takes place slowly over a cycle of 26,000 years.

https://www.youtube.com/watch?v=q4_-R1vnJyw&w=420&h=315

Because the Babylonians kept records dating back millennia, the Greeks were able to formulate their ideas of the truth.

He gave a value for the annual precession of around 46 seconds of arc (compared to a modern figure of 50.26 seconds). He concluded that the whole star pattern was moving slowly eastwards and that it would revolve once every 26,000 years.

Hipparchus also made observations and calculations to determine the orbit of the moon, the dates of eclipses and devised the scale of magnitude or brightness that, considerably amended, is still in use.

PTOLEMY cited Hipparchus as his most important predecessor.

NEXT

Related sites

• From Hipparchus to Hipparcos (wwwhip.obspm.fr/)
• Hipparchus of Rhodes (history.mcs.st-andrews.ac.uk/Biographies/)
• Greek Astronomy (quantumredpill.wordpress.com)

ASTROLOGY

– throughout the Middle Ages, astrology and astronomy were closely linked in both the Western and the Arabic worlds.
Although astrology was used for prediction, pre-modern astrology required a substantial command of mathematics and an informed astronomical knowledge.

PTOLEMY – ‘ The Almagest ’ how the planets move; ‘ Tetrabiblos ’ what effect the qualities of the planets (Mars – hot & dry, Moon – cold & wet [affect on the tides]) and their relative positions will have.

Belief that the influence of the planets may have an effect on earthly health and other matters (disease and character traits).

Tables of positions of planets became developed from the Babylonian originals in the Islāmic world.

Alphonsine tables produced for King Alphonso X of Castile in 1275.

Prognostication repeatedly condemned by the Church as influence of the planets denies the concept of free will.

Refutation of astrology is difficult owing to its complexity.

NEXT

THE MEDIEVAL ARAB SCIENTISTS

A great deal of what we know about the ancient world and its scientific ideas has come to us from documents which were translated from ancient Greek or other ancient languages into Arabic, and later from Arabic into European languages. The material reached the Arab world in many cases through the Roman empire in the East, Byzantium, which survived until 1453, almost a thousand years after the fall of Rome, during the period known in Europe as the Dark Ages.
During this time the consolidating influence of Islāmic religion saw Arab Muslims begin to build an empire that was to stretch across the Middle East and across North Africa into Spain. At the heart of the Islāmic world the caliphs ruled in Baghdad. Arab scientists sowed the seeds that would later be reaped in the scientific revolution of the seventeenth century, especially under the Abbasid dynasty during the caliphate of Harun al-Rashid and his son al-Mamun, and the Middle East became the intellectual hub of the World.

In the ninth century, at the House of Wisdom – a mixture of library, research institute and university – scholars worked to translate the great works of the GREEK thinkers. Muslim scholars of this golden age made important and original contributions to mathematics and astronomy, medicine and chemistry. They developed the ASTROLABE, which enabled astronomers to measure the position of the stars with unparalleled accuracy.
In medicine they made the first serious studies of drugs and advanced surgery. A number of mathematicians, including Habash al-Hasib (‘he who calculates’), Abul’l-Wafa al-Buzjani, Abu Nasr al-Iraq and Ibn Yunus formulated trigonometry (including all six trig functions [ sin, cosec, cos, sec, tan, and cot ]) at a level far above that introduced by the Greek astronomer-mathematician HIPPARCHUS in the second century BCE.
It is largely through such efforts that Greek ideas were preserved through the DARK AGES.

more

Eight hundred years before COPERNICUS, a model of the solar system was advanced with the Earth as a planet orbiting the Sun along with other planets.

A few centuries later this idea fell into disfavour with the early Christian Church, which placed mankind at the centre of the universe in a geo-centric model. The alternative teaching would be deemed heresy punishable by death and it would not be until the seventeenth century that the work of GALILEO, KEPLER and NEWTON gave credence to the ideas revitalized by Copernicus in 1543.

It is worth noting that even to-day at least half the named stars in the sky bear Arabic names (Aldebaran and Algol amongst others) and many terms used in astronomy, such as Nadir and Azimuth, are originally Arabic words.

The elaborate observatory established by the Ulugh Begg in Samarkand in the fifteenth century appeared to function with a dictum meant to challenge PTOLEMY’s geocentric picture of the universe sanctioned by the Church in Europe. Arabic scholars had access to the early teachings of ARISTARCHUS, the astronomer from Samos of the third-century BCE. (referred to by Copernicus in the forward of an early draft of De Revolutionibus, although omitted from the final copy)

NEXT

AL-KHWARIZMI (800-847)

820 – Baghdad, Iraq

The man often credited with the introduction of ‘Arabic’ numerals was al-Khwarizmi, an Arabian mathematician, geographer and astronomer. Strictly speaking it was neither invented by al-Khwarizmi, nor was it Middle Eastern in origin

786 – Harun al-Rashid came to power. Around this time al-Khwarizmi born in Khwarizm, now Khiva, in Uzbekistan.

813 – Caliph al-Ma’mun, the patron of al-Khwarizmi, begins his reign in Baghdad.

Arabic notation has its roots in India around 500 AD, thus the current naming as the ‘Hindu-Arabic’ system. al-Khwarizmi, a scholar in the Dar al-ulum (House of Wisdom) in Baghdad in the ninth century, is responsible for introducing the numerals to Europe. The method of using only the digits 0-9, with the value assigned to them determined by their position, as well as introducing a symbol for zero, revolutionised mathematics.

al-Khwarizmi explained how this system worked in his text ‘Calculation with Hindu numerals‘. He was clearly building upon the work of others before him, such as DIOPHANTUS and BRAHMAGUPTA, and on Babylonian sources that he accessed through Hebrew translations. By standardizing units, Arabic numerals made multiplication, division and every other form of mathematical calculation much simpler. His text ‘al-Kitab al-mukhtasar- fi hisab al-jabr w’al-muqabala’ (The Compendious Book on Calculating by Completion and Balancing) gives us the word algebra. In this treatise, al-Khwarizmi provides a practical guide to arithmetic.

In his introduction to the book he says the aim of the work is to introduce ‘what is easiest and most useful in mathematics, such as men constantly require in cases of inheritance, legacies, partition, lawsuits and trade, and in all their dealings with one another, or when measuring lands, digging canals and making geometrical calculations.’ He introduced quadratic equations, although he described them fully in words and did not use symbolic algebra.
It was in his way of handling equations that he created algebra.

The two key concepts were the ideas of completion and balancing of equations. Completion (al-jabr) is the method of expelling negatives from an equation by moving them to the opposite side

4x² = 54x – 2x²  becomes  6x² = 54x

Balancing (al-muqabala) meanwhile, is the reduction of common positive terms on both sides of the equation to their simplest forms

x² + 3x + 22 = 7x + 12  becomes  x² + 10 = 4x

Thus he was able to reduce every equation to simple, standard forms and then show a method of solving each, showing geometrical proofs for each of his methods – hence preparing the stage for the introduction of analytical geometry and calculus in the seventeenth century.

The name al-Khwarizmi also gives us the word algorithm meaning ‘a rule of calculation’, from the Latin title of the book, Algoritmi de numero Indorum.

NEXT

OMAR KHAYYAM (c.1048–1131)

In the service of the Kurdish-Turkish Sultan Salah al-Din ibn Ayyub (Saladin صلاح الدين يوسف بن أيوب‎ ), the nemesis of Richard the Lionheart during the second crusade, there was published a definitive treatise by Khayyām on algebra in which he classified algebraic equations up to the third degree and showed how geometric solutions to the equations could be obtained

Ironically, the source of Khayyām’s most enduring legacy is neither his mathematics nor his science but rather his poetry. The Rubaiyat, a translation, or recomposition, published initially in 1859 by the British poet Edward Fitzgerald, presents his work in a series of melancholic ruminations concerning the irreversibility of fate and the fleeting nature of life.

One explanation of the decline of science in Islāmic civilization, which began in the late fifteenth century, is the general fatalism that pervaded Islāmic culture, as revealed in the melancholia and pathos of Khayyām’s quatrains describing life continuing among the ruins of ancient grandeur.
Another explanation is the emergence in twelfth century Baghdad of an intellectual movement spearheaded by the fundamentalist al-Ghazali, which favoured faith and dogma over reason and direct evidence.

NEXT

LEONARDO FIBONACCI (c.1170-c.1250)

Also known as Leonardo Pisano. Published ‘Liber Abaci’ in 1202.

1202 – Italy

A series of numbers in which each successive term is the sum of the preceding two

FIBONACCI

For example:   1 , 1 , 2 , 3 , 5 , 8 , 13 , 21 , 34 , 55 , 89 , 144….

The series is known as the Fibonacci sequence and the numbers themselves as the Fibonacci numbers.

The Fibonacci sequence has other interesting mathematical properties – the ratio of successive terms ( larger to smaller;   1/1, 2/1, 3/2, 5/3, 8/5…. ) approaches the number 1.618
This is known as the golden ratio and is denoted by the Greek letter Phi.

Phi was known to ancient Greeks.
Greek architects used the ratio 1:Phi as part of their design, the most famous of which is the Parthenon in Athens.

Phi also occurs in the natural world.
Flowers often have a Fibonacci number of petals.

During his travels in North Africa, Fibonacci learned of the decimal system of numbers that had evolved in India and had been taken up by the Arabs.
In his book Liber Abaci he re-introduced to Europe the Arabic numerals that we use today, adhering roughly to the recipe ‘the value represented must be proportional to the number of straight lines in the symbol’.

Following the Arabs, Fibonacci ( ‘son of the innocent’ – or ‘son of the simpleton’ euph. ) introduced the place–value concept, with each position representing a different power of ten and these arranged in ascending order from right to left.

NEXT

Related sites

• Fibonacci (maths.surrey.ac.uk)
• Evansville facullty (faculty.evansville.edu/ck6/bstud/fibo)

JOHN DEE (1527-1608)

1590 – London, England

JOHN DEE

Mathematician, cartographer & astronomer.
Prolific author, natural magician, alchemist

‘Alternative knowledge and methods of learning. ‘Conversations with Angels’. Human power over the world (neo-Platonism).’

Dee was a Hermetic philosopher, a major influence on the ROSICRUCIANS, possibly a spy – astrologer and adviser to Queen Elizabeth I ; he chose the day of her coronation.

One of the greatest scholars of his day. His library in his home in Mortlake, London, contained more than 3,000 books.

Greatly influenced by Edward Kelley (1555- 97), whom he met in 1582; from 1583-1589 Dee and Kelley sought the patronage of assorted mid-European noblemen and kings, eventually finding it from the Bohemian Count Vilem Rosenberg.

In 1589, Dee left Kelley to his alchemical research and returned to England where Queen Elizabeth I granted him a position as a college warden, but he had lost respect owing to his occult reputation.
Dee returned to Mortlake in 1605 in poor health and increasing poverty and ended his days as a common fortune-teller.

TIMELINE